Section 4.2

Let \(y=f(x)\) be a plot of some graph. Then \(f(x-h)\) will be the plot of \(y=f(x)\) but with a horizontal shift of \(h\) units.

{prf:example}

label:

hozShiftSine1

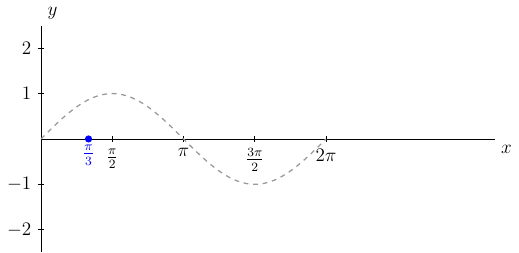
Plot \(y=\sin(x-\frac{\pi}{3})\)

{dropdown} Solution:

First, we see that the magnitude is \(1\).

Second, we say \(\sin(x-\frac{\pi}{3})=\sin(1(x-\frac{\pi}{3}))\), \(B=1\), and using \(\frac{2\pi}{B}\) we see the period is

\[P=\frac{2\pi}{B}=\frac{2\pi}{1}=2\pi\]

Third, given \(\sin(1(x-\frac{\pi}{3}))\) we know that the phase shift is right \(\frac{\pi}{3}\) units.

This means the graph of \(y=\sin(x-\frac{\pi}{3})\) over one period will be over the interval \([0+\frac{\pi}{3},2\pi+\frac{\pi}{3}]=[\frac{\pi}{3},\frac{7\pi}{3}]\). Next, we want to split the period interval into four equal length subintervals. To do this we first find

\[\Delta x = \frac{2\pi}{4}=\frac{\pi}{2}\]

and

\[x_i=x_0+i\Delta x\]

)

Since we are starting at \(x_0=\frac{\pi}{3}\), we know that \(x_1=x_0+1\Delta x\). Then we will do this up to \(x_4\). That is,

\[\begin{align*} x_0 & = \frac{\pi}{3} + 0 \cdot (\frac{\pi}{2})=\frac{\pi}{3}\\ x_1 & = \frac{\pi}{3} + 1 \cdot (\frac{\pi}{2})=\frac{5\pi}{6}\\ x_2 & = \frac{\pi}{3} + 2 \cdot (\frac{\pi}{2})=\frac{4\pi}{3}\\ x_3 & = \frac{\pi}{3} + 3 \cdot (\frac{\pi}{2})=\frac{11\pi}{6}\\ x_4 & = \frac{\pi}{3} + 4 \cdot (\frac{\pi}{2})=\frac{7\pi}{3}\\ \end{align*}\]